1.

Introduction

The angular momentum of an electromagnetic wave consists of the spin angular momentum manifested through the polarization state and the orbital angular momentum manifested through the spatial distribution of the components of the electric and the magnetic fields.¹ Both parts of the angular momentum are mechanically equivalent and can be used to rotate a trapped particle.² Spin angular momentum can be converted to orbital angular momentum in a nonhomogeneous anisotropic material.³ Beams of light with nonzero orbital angular momentum find applications for optical micromanipulation, quantum information encoding, and control of atoms.⁴

Surface-plasmon-polariton (SPP) waves are the surface electromagnetic waves guided by an interface of a metal and a dielectric material. The electromagnetic field of an SPP wave is confined to the vicinity of the metal/dielectric interface. This confinement enhances the intensity of the electric field and, thus, leads to the high sensitivity of SPP waves to surface conditions. The high sensitivity is commonly exploited in SPP-based chemical and biosensing devices.⁵ SPP waves are also exploited in single-molecule detectors,⁶ optical nanoantennas,^6,7 low-loss plasmonic waveguides,⁸ and integrated optical circuits.⁹

The angular momentum of SPP waves has not been widely investigated,¹⁰ even though some studies have suggested the potential for applications (1) in communications, due to the independence of the spin and the orbital parts of angular momentum;¹¹ (2) for molecular sensing, due to the conservation of total angular momentum;¹⁰ and (3) in tunable nanosized plasmonic motors, due to the enhancement of the electric field in the dielectric partnering material near the interface with the metallic partnering material.¹²

At a given frequency, only one SPP wave—that too of $p$-polarization state—can be guided by a metal/dielectric interface, if the dielectric material is homogeneous.⁵ The fixed polarization state restricts the choices for the direction of the angular momentum of the SPP wave. With just a single SPP wave available, there is not much room to maneuver the magnitude of the angular momentum either.

During the last 5 years, it has been established that multiple SPP waves of the same frequency but with different phase speed, attenuation rate, and spatial field profiles can be guided by the planar interface of a metal and a periodically nonhomogeneous dielectric material—whether that dielectric material is isotropic^13–15 or anisotropic^16–21—the direction of periodic nonhomogeneity being normal to the interface plane. For example, the partnering dielectric material can be a rugate filter with a sinusoidally varying refractive index,¹³ a periodically nonhomogeneous sculptured nematic thin film (SNTF),^16–20 or a chiral sculptured thin film.²¹ These developments have spurred the concept of surface multiplasmonics,²² with potential applications for multianalyte optical sensors²³ and plasmonic solar cells.²⁴

The present series of papers is devoted to surface multiplasmonics supported by the planar interface of a metal and an SNTF that was fabricated by sinusoidal rocking of a substrate-holding platform during physical vapor deposition in a vacuum chamber. Parts I–III^16–18 showed theoretically and experimentally that multiple SPP waves—that differ in phase speed, attenuation rate, and spatial field profiles, but not in frequency and direction of propagation—can be guided by the metal/SNTF interface in the prism-coupled configuration commonly employed to launch and exploit SPP waves.⁵ Part IV¹⁹ was devoted to the underlying canonical boundary-value problem, and Part V²⁰ delineated the surface multiplasmonics phenomenon in the grating-coupled configuration. As the availability of multiple SPP waves with different spatial field distributions may offer a wide range of possibilities for the magnitudes and directions of spin and orbital angular momentums, in this article—labeled as Part VI—we set out to investigate the angular momentums of the SPP waves guided by the metal/SNTF interface in the canonical boundary-value problem.

The formulation of the canonical boundary-value problem is revisited in brief in Sec. 2.1, and the methodology to compute the spin and orbital angular momentums is provided in Sec. 2.2. Numerical results are presented and discussed in Sec. 3 when the partnering dielectric material is (1) an isotropic and homogeneous dielectric material; (2) an isotropic dielectric material whose refractive index varies sinusoidally in the direction normal to the interface plane; (3) a columnar thin film (CTF), which is an anisotropic and homogeneous dielectric material; or (4) a periodically nonhomogeneous SNTF of the type considered in Parts I–V. The article concludes with a discussion in Sec. 4.

An $\exp (-i\omega t)$ time-dependence is implicit with $\omega$ denoting the angular frequency and $t$ being time. The free-space wavenumber, the free-space wavelength, and the intrinsic impedance of free space are denoted by $k_0=\omega \sqrt{{ϵ}_0{\mu }_0}$, ${\lambda }_0=2\pi /k_0$, and ${\eta }_0=\sqrt{{\mu }_0/{ϵ}_0}$, respectively, with ${\mu }_0$ and ${ϵ}_0$ being the permeability and the permittivity of free space, respectively. Vectors are in boldface, dyadics are underlined twice, column vectors are in boldface and enclosed within square brackets, and matrixes are underlined twice and square bracketed. The asterisk denotes the complex conjugate, and the Cartesian unit vectors are identified as ${\widehat{\mathbf{u}}}_x$, ${\widehat{\mathbf{u}}}_y$, and ${\widehat{\mathbf{u}}}_z$.

2.

Theory

3.

Numerical Results and Discussion

For all the numerical results presented in this section, the free-space wavelength was fixed at ${\lambda }_0=633\mathrm{nm}$, and the metal was taken to be bulk aluminum: ${\epsilon }_{\mathrm{met}}=-56+21i$. We normalized ${\mathbf{M}}_s^{\mathrm{Mink}}$, etc., to

The integrals over $z\in (-\infty ,\infty )$ on the right sides of Eqs. (11)–(15) were evaluated analytically for the data reported in Sec. 3.1. For the data provided in Secs. 3.2–3.4, these integrals were interpreted as follows:

3.3.

Homogeneous Anisotropic Partnering Dielectric Material

Before we proceed to the metal/SNTF interface, let us consider the interface of a metal and a CTF. A CTF is a homogeneous biaxial dielectric material with a relative permittivity dyadic³³

Eq. (22)

$${\underset{=}{ϵ}}_{\mathrm{diel}}(z)={\underset{=}{S}}_z(\gamma )·{\underset{=}{s}}_y(\chi )·{\underset{=}{ϵ°}}_{\mathrm{ref}}·{\underset{=}{s}}_y^{-1}(\chi )·{\underset{=}{S}}_z^{-1}(\gamma ),$$

where the dyadics

Eq. (23)

$$\begin{array}{l}{\underset{=}{s}}_y(\chi )=({\widehat{\mathbf{u}}}_x{\widehat{\mathbf{u}}}_x+{\widehat{\mathbf{u}}}_z{\widehat{\mathbf{u}}}_z)\cos \chi +({\widehat{\mathbf{u}}}_z{\widehat{\mathbf{u}}}_x-{\widehat{\mathbf{u}}}_x{\widehat{\mathbf{u}}}_z)\sin \chi +{\widehat{\mathbf{u}}}_y{\widehat{\mathbf{u}}}_y\\ {\underset{=}{ϵ°}}_{\mathrm{ref}}={ϵ}_a{\widehat{\mathbf{u}}}_z{\widehat{\mathbf{u}}}_z+{ϵ}_b{\widehat{\mathbf{u}}}_x{\widehat{\mathbf{u}}}_x+{ϵ}_c{\widehat{\mathbf{u}}}_y{\widehat{\mathbf{u}}}_y\end{array}\}$$

depend on the inclination $\chi$ of the columns of the CTF. The third dyadic on the right side of Eq. (22) is stated as

Eq. (24)

$${\underset{=}{S}}_z(\gamma )=({\widehat{\mathbf{u}}}_x{\widehat{\mathbf{u}}}_x+{\widehat{\mathbf{u}}}_y{\widehat{\mathbf{u}}}_y)\cos \gamma +({\widehat{\mathbf{u}}}_y{\widehat{\mathbf{u}}}_x-{\widehat{\mathbf{u}}}_x{\widehat{\mathbf{u}}}_y)\sin \gamma +{\widehat{\mathbf{u}}}_z{\widehat{\mathbf{u}}}_z,$$

so that the morphologically significant plane of the CTF is formed by the unit vectors ${\widehat{\mathbf{u}}}_z$ and ${\widehat{\mathbf{u}}}_x\cos \gamma +{\widehat{\mathbf{u}}}_y\sin \gamma$, where $\gamma$ is the angle between the morphologically significant plane and the direction of propagation of SPP waves. For a CTF made by evaporating patinal titanium oxide, let us use³⁴

Eq. (25)

$$\begin{array}{l}{ϵ}_a={(1.0443+2.7394v-1.3697v^2)}^2\\ {ϵ}_b={(1.6765+1.5649v-0.7825v^2)}^2\\ {ϵ}_c={(1.3586+2.1109v-1.0554v^2)}^2\\ \chi ={\tan }^{-1}(2.8818\tan {\chi }_v)\end{array}\},$$

where $v=2{\chi }_v/\pi$ and ${\chi }_v=45\mathrm{deg}$, for a comparison with the numerical results for a metal/SNTF interface provided in Sec. 3.4.

Only one SPP wave can be guided by the metal/CTF interface, irrespective of the angle $\gamma$. The real and imaginary parts of the relative wavenumbers $q/k_0$ obtained by the solution of the dispersion equation [Eq. (10)] are provided in Fig. 1 as functions of $\gamma \in [0\mathrm{deg},90\mathrm{deg}]$. The SPP wave is neither $p$- nor $s$-polarized, except when $\gamma =0\mathrm{deg}$.

Fig. 1

The real and imaginary parts of relative wavenumbers $q/k_0$ as functions of $\gamma$ for SPP waves guided by a metal/CTF interface. The metal is bulk aluminum, whereas the chosen CTF is described in Sec. 3.3.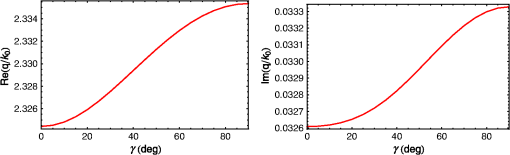

3.3.1.

$\gamma \mathsf{=}\mathsf{0}\mathsf{deg}$

When $\gamma =0\mathrm{deg}$, the SPP wave is $p$-polarized with $q/k_0=2.3244+0.03261i$. For this SPP wave, we found that

Eq. (26)

$${\mathbf{m}}_s^{\mathrm{Mink}}=-1.51\times {10}^{-10}{\widehat{\mathbf{u}}}_y\mathrm{Nm}/\mathrm{F},{\mathbf{m}}_{\mathrm{o}}^{\mathrm{Mink}}=1.87\times {10}^{-9}{\widehat{\mathbf{u}}}_y\mathrm{Nm}/\mathrm{F},$$

and

Eq. (27)

$${\mathbf{m}}_s^{\mathrm{Abr}}=-2.97\times {10}^{-11}{\widehat{\mathbf{u}}}_y\mathrm{Nm}/\mathrm{F},{\mathbf{m}}_{\mathrm{o}}^{\mathrm{Abr}}=2.32\times {10}^{-10}{\widehat{\mathbf{u}}}_y\mathrm{Nm}/\mathrm{F},$$

with $a_p=1\mathrm{V}/\mathrm{m}$ and $a_s=0$. As in the case with isotropic partnering dielectric materials in Secs. 3.1 and 3.2, the angular momentum of the SPP wave lies wholly in the interface plane and is oriented perpendicular to the direction of the propagation in both the Minkowski and Abraham formulations. Moreover, in both formulations, with a small spin part opposing a large orbital part, the angular momentum is predominantly orbital in nature. Furthermore, $|{\mathbf{m}}_s^{\mathrm{Mink}}|>|{\mathbf{m}}_s^{\mathrm{Abr}}|$ and $|{\mathbf{m}}_{\mathrm{o}}^{\mathrm{Mink}}|>|{\mathbf{m}}_{\mathrm{o}}^{\mathrm{Abr}}|$ by an order of magnitude.

3.3.2.

$\mathsf{\gamma }\mathsf{\in }\mathsf{(}\mathsf{0}\mathsf{deg},\mathsf{90}\mathsf{deg}\mathsf{]}$

For $\gamma \in (0\mathrm{deg},90\mathrm{deg}]$, the Cartesian components of ${\mathbf{m}}_{s,\mathrm{o}}^{\mathrm{Mink},\mathrm{Abr}}$ are plotted in Fig. 2 for the sole SPP wave guided by the metal/CTF interface. All Cartesian components of ${\mathbf{m}}_{s,\mathrm{o}}^{\mathrm{Mink},\mathrm{Abr}}$ are nonzero. Furthermore, the Cartesian components of the spin angular momentum are smaller than their orbital counterparts in both the Minkowski and Abraham formulations.

Fig. 2

The Cartesian components of (a) ${\mathbf{m}}_{\mathrm{o}}^{\mathrm{mink}}$, (b) ${\mathbf{m}}_s^{\mathrm{Mink}}$, (c) ${\mathbf{m}}_{\mathrm{o}}^{\mathrm{Abr}}$, and (d) ${\mathbf{m}}_s^{\mathrm{Abr}}$ as functions of $\gamma$ for SPP waves guided by the interface of bulk aluminum and the chosen titanium-oxide CTF when $a_p=1\mathrm{V}/\mathrm{m}$. The $x$-, $y$-, and $z$-directed components are represented by red solid, blue dashed, and black chain-dashed lines, respectively.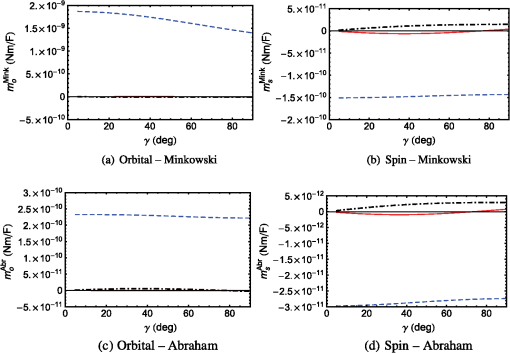

A scan of Fig. 2(a) shows that the magnitude of the $y$-directed component of ${\mathbf{m}}_{\mathrm{o}}^{\mathrm{Mink}}$ is significantly larger than the magnitudes of its $x$- and $z$-directed components; therefore, ${\mathbf{m}}_{\mathrm{o}}^{\mathrm{Mink}}$ is predominantly directed along an axis ($y$-axis) that lies wholly in the interface plane and is oriented perpendicular to the direction of propagation. Similarly, a scan of Fig. 2(c) shows that ${\mathbf{m}}_{\mathrm{o}}^{\mathrm{Abr}}$ is also oriented very similarly. But, ${\mathbf{m}}_s^{\mathrm{Mink}}$ and ${\mathbf{m}}_s^{\mathrm{Abr}}$ in Figs. 2(b) and 2(d), respectively, are oriented along the $-y$-axis.

A comparison of Figs. 2(a) and 2(b) with Figs. 2(c) and 2(d) shows that the magnitudes of the Cartesian components of the Minkowski orbital and spin angular momentums generally exceed those of their Abraham counterparts.

The magnitudes of ${\mathbf{m}}_{s,\mathrm{o}}^{\mathrm{Mink},\mathrm{Abr}}$ as functions of $\gamma \in (0\mathrm{deg},90\mathrm{deg}]$ are presented in Fig. 3. The spin angular momentum is weaker than the orbital angular momentum in both the Minkowski and Abraham formulations. Furthermore, $|{\mathbf{m}}_s^{\mathrm{Mink}}|>|{\mathbf{m}}_s^{\mathrm{Abr}}|$ and $|{\mathbf{m}}_{\mathrm{o}}^{\mathrm{Mink}}|>|{\mathbf{m}}_{\mathrm{o}}^{\mathrm{Abr}}|$.

Fig. 3

The magnitudes of ${\mathbf{m}}_{s,\mathrm{o}}^{\mathrm{Mink},\mathrm{Abr}}$ as functions of $\gamma$ for SPP waves guided by the interface of bulk aluminum and the chosen titanium-oxide CTF when $a_p=1\mathrm{V}/\mathrm{m}$. Results for the Abraham and the Minkowski formulations are denoted by red solid and blue dashed lines, respectively.

3.4.

Periodically Nonhomogeneous Anisotropic Partnering Dielectric Material

Let us now consider that the half-space $z>0$ is occupied by an SNTF with a periodically nonhomogeneous relative permittivity dyadic²⁰

Eq. (28)

$${\underset{=}{ϵ}}_{\mathrm{diel}}(z)={\underset{=}{S}}_z(\gamma )·{\underset{=}{S}}_y(z)·{\underset{=}{ϵ°}}_{\mathrm{ref}}(z)·{\underset{=}{S}}_y^{-1}(z)·{\underset{=}{S}}_z^{-1}(\gamma ),$$

where the dyadics

Eq. (29)

$$\begin{array}{l}{\underset{=}{S}}_y(z)=({\widehat{\mathbf{u}}}_x{\widehat{\mathbf{u}}}_x+{\widehat{\mathbf{u}}}_z{\widehat{\mathbf{u}}}_z)\cos [\chi (z)]+({\widehat{\mathbf{u}}}_z{\widehat{\mathbf{u}}}_x-{\widehat{\mathbf{u}}}_x{\widehat{\mathbf{u}}}_z)\sin [\chi (z)]+{\widehat{\mathbf{u}}}_y{\widehat{\mathbf{u}}}_y\\ {\underset{=}{ϵ°}}_{\mathrm{ref}}(z)={ϵ}_a(z){\widehat{\mathbf{u}}}_z{\widehat{\mathbf{u}}}_z+{ϵ}_b(z){\widehat{\mathbf{u}}}_x{\widehat{\mathbf{u}}}_x+{ϵ}_c(z){\widehat{\mathbf{u}}}_y{\widehat{\mathbf{u}}}_y\end{array}\}$$

depend on the sinusoidally varying vapor incidence angle

Eq. (30)

$${\chi }_v(z)={\tilde{\chi }}_v+{\delta }_v\sin (\pi z/\mathrm{\Omega }).$$

As the third dyadic on the right side of Eq. (28) is given by Eq. (24), the morphologically significant plane of the SNTF is also formed by the unit vectors ${\widehat{\mathbf{u}}}_z$ and ${\widehat{\mathbf{u}}}_x\cos \gamma +{\widehat{\mathbf{u}}}_y\sin \gamma$.

Following Parts I, II, IV, and V, an SNTF made by evaporating patinal titanium oxide³⁴ was considered with

Eq. (31)

$$\begin{array}{l}{ϵ}_a(z)={[1.0443+2.7394v(z)-1.3697v^2(z)]}^2\\ {ϵ}_b(z)={[1.6765+1.5649v(z)-0.7825v^2(z)]}^2\\ {ϵ}_c(z)={[1.3586+2.1109v(z)-1.0554v^2(z)]}^2\\ \chi (z)={\tan }^{-1}[2.8818\tan {\chi }_v(z)]\end{array}\},$$

where $v(z)=2{\chi }_v(z)/\pi$. The following quantities were fixed for all numerical results presented here: ${\tilde{\chi }}_v=45\mathrm{deg}$, ${\delta }_v=30\mathrm{deg}$, and $\mathrm{\Omega }=200\mathrm{nm}$. Let us note that the CTF considered in Sec. 3.3 can be treated as a special case of the SNTF considered in this section with ${\delta }_v=0\mathrm{deg}$.

The real and imaginary parts of the relative wavenumbers $q/k_0$ obtained by the solution of the dispersion equation [Eq. (10)] are provided in Fig. 4 as functions of $\gamma$. These solutions have been reproduced from Part IV.¹⁹ Either two or three SPP waves can be guided by the metal/SNTF interface, depending on the angle $\gamma$ between the direction of propagation and the morphologically significant plane of the SNTF. The SPP waves guided by the metal/SNTF interface are neither $p$- nor $s$-polarized, except when $\gamma =0\mathrm{deg}$.

Fig. 4

The real and imaginary parts of relative wavenumbers $q/k_0$ as functions of $\gamma$ for SPP waves guided by a metal/SNTF interface.¹⁹ The metal is bulk aluminum, whereas the chosen SNTF is described in Sec. 3.4.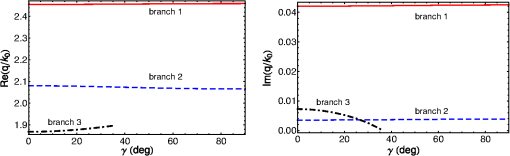

3.4.1.

$\mathsf{\gamma }\mathsf{=}\mathsf{0}\mathsf{deg}$

When $\gamma =0\mathrm{deg}$, two $p$- and one $s$-polarized SPP waves can be guided by the metal/SNTF interface. The values of the relative wavenumber $q/k_0$ and the $y$-components of ${\mathbf{m}}_{s,\mathrm{o}}^{\mathrm{Mink},\mathrm{Abr}}$ are provided in Table 2.

Table 2

Relative wavenumbers q/k0 and y-directed components of ms,oMink,Abr of SPP waves guided by the interface of bulk aluminum and the chosen titanium-oxide SNTF when γ=0  deg and λ0=633  nm. The values of Nd used in Eq. (18) are also provided. The x- and z-directed components of ms,oMink,Abr are identically zero. The computations were made by setting {ap=1,as=0}  V/m for p-polarized SPP waves and {ap=0,as=1}  V/m for s-polarized SPP waves.

Polarization state	$q/k_0$	$N_d$	Normalized spin angular momentum (Nm/F)	Normalized orbital angular momentum (Nm/F)
$p$ (branch 1)	$2.4550+0.0421i$	2	$m_{\mathrm{sy}}^{\mathrm{Mink}}=-1.09\times {10}^{-10}$	$m_{\mathrm{oy}}^{\mathrm{Mink}}=8.30\times {10}^{-10}$
			$m_{\mathrm{sy}}^{\mathrm{Abr}}=-1.88\times {10}^{-11}$	$m_{\mathrm{oy}}^{\mathrm{Abr}}=1.02\times {10}^{-10}$
$s$ (branch 2)	$2.080+0.0035i$	6	$m_{\mathrm{sy}}^{\mathrm{Mink}}=0$	$m_{\mathrm{oy}}^{\mathrm{Mink}}=2.75\times {10}^{-9}$
			$m_{\mathrm{sy}}^{\mathrm{Abr}}=0$	$m_{\mathrm{oy}}^{\mathrm{Abr}}=4.34\times {10}^{-10}$
$p$ (branch 3)	$1.8683+0.0073i$	10	$m_{\mathrm{sy}}^{\mathrm{Mink}}=-8.63\times {10}^{-10}$	$m_{\mathrm{oy}}^{\mathrm{Mink}}=1.59\times {10}^{-7}$
			$m_{\mathrm{sy}}^{\mathrm{Abr}}=-2.55\times {10}^{-10}$	$m_{\mathrm{oy}}^{\mathrm{Abr}}=2.03\times {10}^{-8}$

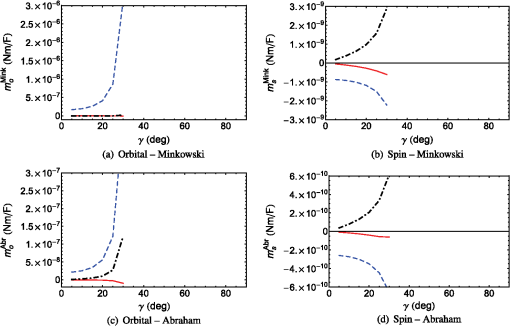
For both $p$-polarized SPP waves, ${\mathbf{m}}_s$ and ${\mathbf{m}}_{\mathrm{o}}$ are anti-parallel in both the Minkowski and Abraham formulations. Furthermore, both parts of the angular momentum are directed along an axis that lies wholly in the interface plane and is oriented perpendicular to the direction of propagation—in accord with a $p$-polarized SPP wave guided by either a metal/isotropic-dielectric or a metal/CTF interface when $\mathrm{Re}\{q/k_0\}>1$. For the only $s$-polarized SPP wave, ${\mathbf{m}}_s$ is identically zero in both the Minkowski and Abraham formulations.

As was found for the interface of a metal and an isotropic dielectric partnering material—whether homogeneous (Sec. 3.1) or periodically nonhomogeneous (Sec. 3.2)—the magnitudes of the Minkowski spin and orbital angular momentums are higher than those of their Abraham counterparts; furthermore, the magnitudes of ${\mathbf{m}}_{s,\mathrm{o}}^{\mathrm{Mink},\mathrm{Abr}}$ increase significantly as the phase speed $\omega /\mathrm{Re}\{q\}$ of the SPP wave increases.

3.4.2.

$\mathsf{\gamma }\mathsf{\in }\mathsf{(}\mathsf{0}\mathsf{deg},\mathsf{90}\mathsf{deg}\mathsf{]}$

For $\gamma \in (0\mathrm{deg},90\mathrm{deg}]$, the Cartesian components of ${\mathbf{m}}_{\mathrm{o},s}^{\mathrm{Mink},\mathrm{Abr}}$ are plotted in Fig. 5 for the SPP waves guided by the chosen metal/SNTF interface for the solutions on branch 1 in Fig. 4. The $y$-directed component of ${\mathbf{m}}_{\mathrm{o}}^{\mathrm{Mink}}$ is greater in magnitude than the other components of ${\mathbf{m}}_{\mathrm{o}}^{\mathrm{Mink}}$; therefore, ${\mathbf{m}}_{\mathrm{o}}^{\mathrm{Mink}}$ can be said to lie in the interface plane and oriented perpendicular to the direction of propagation. A similar remark can also be made for ${\mathbf{m}}_s^{\mathrm{Mink}}$, ${\mathbf{m}}_{\mathrm{o}}^{\mathrm{Abr}}$, and ${\mathbf{m}}_s^{\mathrm{Abr}}$, though ${\mathbf{m}}_s$ is directed opposite to ${\mathbf{m}}_{\mathrm{o}}$. A comparison of Figs. 2 and 5 shows that the angular momentum of the SPP wave represented by branch 1 in Fig. 4 for the metal/SNTF interface and for the sole SPP wave guided by the metal/CTF interface are similar in magnitudes and directions, in both formulations.

Fig. 5

The Cartesian components of (a) ${\mathbf{m}}_{\mathrm{o}}^{\mathrm{mink}}$, (b) ${\mathbf{m}}_s^{\mathrm{Mink}}$, (c) ${\mathbf{m}}_{\mathrm{o}}^{\mathrm{Abr}}$, and (d) ${\mathbf{m}}_s^{\mathrm{Abr}}$ as functions of $\gamma$ for solution branch 1 (in Fig. 4) representing the SPP waves guided by the interface of bulk aluminum and the chosen titanium-oxide SNTF when $a_p=1\mathrm{V}/\mathrm{m}$. The $x$-, $y$-, and $z$-directed components are represented by red solid, blue dashed, and black chain-dashed lines, respectively. For computations, $N_d=2$ was set after ascertaining that the values of the Cartesian components of ${\mathbf{m}}_s$ and ${\mathbf{m}}_{\mathrm{o}}$ converged.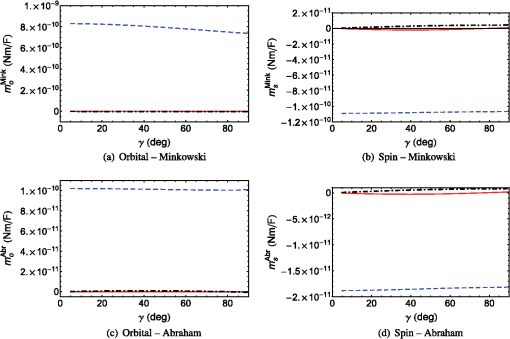

For the SPP waves on branch 2, the Cartesian components of ${\mathbf{m}}_{\mathrm{o},s}^{\mathrm{Mink},\mathrm{Abr}}$ are plotted in Fig. 6 as functions of $\gamma$. A scan of Figs. 6(a) and 6(c) shows that the orbital angular momentum lies almost wholly in the interface plane and is perpendicular to the direction of propagation. However, ${\mathbf{m}}_s^{\mathrm{Mink}}$ lies in the $xz$-plane, because the $x$- and $z$-directed components of ${\mathbf{m}}_s^{\mathrm{Mink}}$ are similar in magnitude and are greater in magnitude than the $y$-directed component [Fig. 6(b)]. Similarly, ${\mathbf{m}}_s^{\mathrm{Abr}}$ also lies in the $xz$-plane. A comparison of Figs. 5 and 6 shows that the Cartesian components of the angular momentums of SPP waves on branch 2 are significantly larger than of those on branch 1.

Fig. 6

Same as Fig. 5 except for solution on branch 2 and $N_d=6$.

The Cartesian components of ${\mathbf{m}}_{\mathrm{o},s}^{\mathrm{Mink},\mathrm{Abr}}$ for branch 3 are presented only for $\gamma \le 30\mathrm{deg}$ in Fig. 7 for convenience, because the magnitude of either one or two components increases rapidly as $\gamma$ increases from 30 deg to 36 deg. Figures 7(a) and 7(c) show that the orbital angular momentum is directed perpendicular to the direction of propagation and lies in the interface plane, because the $y$-directed component is larger in magnitude than the $x$- and $z$-directed components in both formulations. Similarly, Figs. 7(b) and 7(d) show that ${\mathbf{m}}_s^{\mathrm{Mink}}$ and ${\mathbf{m}}_s^{\mathrm{Abr}}$ are directed along the $-y$-axis when $\gamma$ is small and lie in the $yz$-plane when $\gamma$ is large.

Fig. 7

Same as Fig. 5 except for solution on branch 3 and $N_d=10$.

From Figs. 5–7, we conclude that all Cartesian components of ${\mathbf{m}}_s^{\mathrm{Mink}}$ and ${\mathbf{m}}_{\mathrm{o}}^{\mathrm{Mink}}$ are nonzero for $\gamma \in (0\mathrm{deg},90\mathrm{deg}]$, in contrast to the case when $\gamma =0\mathrm{deg}$. The nonzero $x$- and $z$-directed components are also in contrast with the results presented in Secs. 3.1 and 3.2.

The magnitudes of ${\mathbf{m}}_{s,\mathrm{o}}^{\mathrm{Mink},\mathrm{Abr}}$ as functions of $\gamma \in (0\mathrm{deg},90\mathrm{deg}]$ are presented in Fig. 8 for all three branches of solutions in Fig. 4. A quick scan of Fig. 8 shows the availability of a wide range of magnitudes of ${\mathbf{m}}_s$ and ${\mathbf{m}}_{\mathrm{o}}$ in both the Minkowski and Abraham formulations for different SPP waves guided by the metal/SNTF interface. A comparison of Figs. 8(a), 8(c), and 8(e) with Figs. 8(b), 8(d), and 8(f) indicates that the magnitude of the spin angular momentum is lower than that of the orbital angular momentum in both formulations. Moreover, the Minkowski orbital and spin angular momentums are larger in magnitude than their Abraham counterparts. These trends are similar to the cases when partnering dielectric material is either isotropic (Secs. 3.1 and 3.2) or homogeneous and anisotropic (Sec. 3.3).

Fig. 8

The magnitudes of ${\mathbf{m}}_{s,\mathrm{o}}^{\mathrm{Mink},\mathrm{Abr}}$ as functions of $\gamma$ of SPP waves guided by the interface of bulk aluminum and the chosen titanium-oxide SNTF when $a_p=1\mathrm{V}/\mathrm{m}$. The panels (a, b), (c, d), and (e, f) correspond to the solution on branches labeled 1, 2, and 3 in Fig. 4. For computations, $N_d=2$ for branch 1, 6 for branch 2, and 10 for branch 3 were set after ascertaining that the values of the Cartesian components of ${\mathbf{m}}_s$ and ${\mathbf{m}}_{\mathrm{o}}$ converged. Results for the Abraham and Minkowski formulations are denoted by red solid and blue dashed lines, respectively.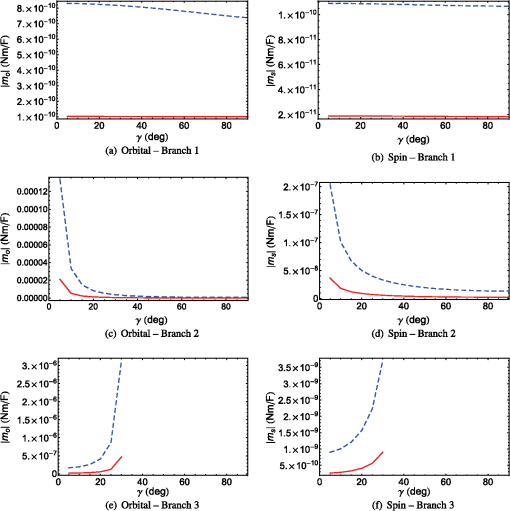

Analysis of Fig. 8 and Tables 1 and 2 shows that the magnitudes of ${\mathbf{m}}_{s,\mathrm{o}}^{\mathrm{Mink},\mathrm{Abr}}$ are generally higher when the SPP waves are neither $p$- nor $s$-polarized, except for the SPP waves on branch 1 guided by the metal/SNTF interface. Parenthetically, we note that the polarization state of the SPP waves on branch 1 is very near to the $p$-polarization state,¹⁹ which may explain the lower magnitudes of the spin and orbital angular momentums for SPP waves on this branch. Of all the SPP waves guided by metal/SNTF interface, the SPP waves on branch 2 generally have the highest magnitudes of the spin and orbital angular momentums in both formulations.

A comparison of the results presented in Sec. 3.3 for the metal/CTF interface and in this section for the metal/SNTF interface shows that:

• • Numerous directions are available for the angular momentums of SPP waves guided by the metal/SNTF interface in contrast to the sole SPP wave guided by the metal/CTF interface.

• • The spin and the orbital angular momentums for the branch 1 in Fig. 4 for the metal/SNTF interface are comparable in magnitude with those for the sole branch in Fig. 1 for the metal/CTF interface.

• • The magnitudes of both spin and orbital angular momentums are higher by at least an order of magnitude for branches 2 and 3 than for branch 1 in Fig. 4 for the metal/SNTF interface.

Let us note that the comparison between the results for the metal/CTF and metal/SNTF interfaces is quite appropriate, because the relative permittivity dyadic of the SNTF can be transformed into the relative permittivity dyadic of the CTF by setting ${\delta }_v=0\mathrm{deg}$. Parenthetically, we note that the conclusions derived by using an SNTF of patinal titanium oxide as the partnering dielectric material should not change qualitatively even if the SNTF were to be considered as made by evaporating another dielectric material, because many of the interesting features of an SPP wave guided by a metal/SNTF interface result from the SPP wave not being linearly polarized.

4.

Concluding Remarks

The time-averaged spin and orbital parts of the angular momentums of multiple SPP waves guided by the interface of a metal and a periodically nonhomogeneous SNTF were theoretically investigated using the solutions of the underlying canonical boundary-value problem. Multiple SPP waves propagate not only with different phase speeds, attenuation rates, and spatial field profiles (in particular, the degree of localization to the interface in the SNTF), but also with different magnitudes and directions of the spin and orbital parts of the angular momentum—calculated using either of the two formulations: one due to Minkowski and the other due to Abraham. The magnitudes and directions of the angular momentums of SPP waves guided by a metal/SNTF interface can vary over large ranges.

For all SPP waves guided by the metal/SNTF interface, the orbital angular momentum lies in the interface plane and is oriented perpendicular to the direction of propagation. For the SPP waves on branch 1, the spin angular momentum is similarly directed. For the SPP waves on branch 2, the spin angular momentum lies in the plane formed by the direction of propagation and normal to the interface; for the SPP waves on branch 3, it is perpendicular to the direction of propagation but does not lie in the interface plane. The magnitude of the spin angular momentum is less than that of the orbital angular momentum, in both formulations. Furthermore, the magnitudes of both the spin and the orbital angular momentums are higher for the SPP waves guided by the metal/SNTF interface (when the propagation of SPP waves does not take place in the morphologically significant plane) than the metal/rugate-filter interface. The magnitudes of the spin and orbital parts of the Minkowski angular momentum were found to be larger than of their Abraham counterparts.

The replacement of an isotropic, homogeneous, partnering dielectric material by an isotropic, periodically nonhomogeneous, partnering dielectric material does not change the direction of the spin and orbital parts of the angular momentums of SPP waves, but the magnitudes are certainly enhanced. At the same time, periodic nonhomogeneity engenders a multiplicity of SPP waves. The replacement of an anisotropic, homogeneous, partnering dielectric material by an anisotropic, periodically nonhomogeneous, partnering dielectric material not only engenders a multiplicity of SPP waves and significantly enhances the magnitudes of the spin and orbital parts of the angular momentums of SPP waves, but it also affords numerous directions for those quantities.

The conclusions on the spin and orbital angular momentum densities obtained here through the solution of the canonical boundary-value problem should hold for the prism-coupled configuration¹⁷ as long as the partnering materials are sufficiently thick in that configuration.¹⁴ However, the results could be somewhat different for the grating-coupled configuration,²⁰ due to the presence of multiple Floquet modes arising from the periodic corrugation of the interface of the partnering materials.

Let us note in closing that the relative permittivity dyadic of the SNTF can be engineered during fabrication.²⁵ Therefore, the magnitudes and the directions of the spin and the orbital parts of the angular momentum can be engineered. This flexibility in the design of SNTFs—not to mention sculptured thin films of other types²⁵—and the choices of the spin and the orbital angular momentums thereby available may have potential for the trapping and rotation of single molecules that infiltrate the partnering SNTF. Furthermore, in microfluidic channels, metal/SNTF microflakes could be used for directed transportation of molecules stowed inside the porous SNTF, the translation and rotation of each microflake effected through a beam of light.